The best references for lie theory are the three volumes lie groups and lie algebras chapters 1 3 lie groups and lie. Indeed the exponential map is locally a diffeomorphism from a neighborhood of the.
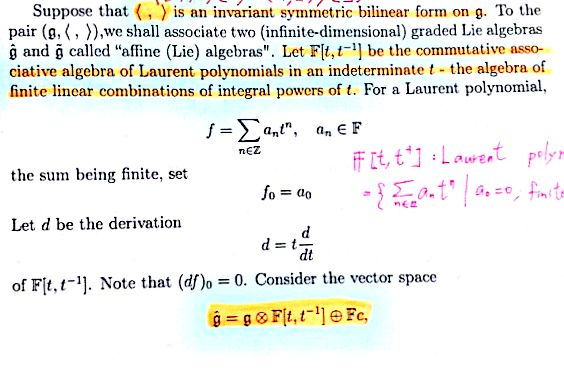Suppose That Is An Invariant Symmetric Bilinear Fo
The lie algebra of v endomorphisms x that satisfy 3 is called o2 1f for orthogonal.
[PDF] Free Download Notes On Lie Algebras Book. L l that takes the bracket in lto the bracket in l ie. Naturally one could generalize the definition by allowing the vector space to be of infinite dimension or by replacing vector space by mod. All matrices of any fixed dimension with trace 0 2 the orthogonal ones i.
φab l φaφb l. Note that o 2 1 f sl 2 1 f. A lie algebra is simple if it has no non trivial ideals and is not abelian.
Universal envelopping algebras levis theorem serres theorem kac moody lie algebra the kostants form of the envelopping algebra and a beginning of a proof of the chevalleys theorem. Cartan sub lie algebra roots weyl group dynkin diagram. Then the local structure of gnear the identity ie.
Suppose that g is the lie algebra of a lie group g. The third chapter brings the theory of the finite dimensional representations of a semisimple lie alge bra with the highest or extreme weight as central notion. The rule for the product of two elements of gsufficiently closed to the identity is determined by its lie algebra g.
There is also a discus sion of the compact form and other real forms of a complex semisimple lie algebra and a section on automorphisms. Root systems and simple lie algebras 123. Lecture notes in lie algebras this note covers the following topics.
Thus any finite group is a 0 dimensional lie group. There are several equivalent characterizations of semisimple algebras such as having no nonzero solvable ideals. Moreover dim o 2 1 f 2 1 the same.
Basic definitions note that the definition of a lie group does not require that g be connected. All skewsymmetric ma trices of any fixed dimension 3 the symplectic ones i. That is to say a one dimensionalnecessarily abelianlie algebra is by definition not simple even though it has no nontrivial ideals a lie algebra is called semisimple if it is isomorphic to a direct sum of simple algebras.
Reference is the book introduction to lie algebras and representation the ory by james e. A lie algebra isomorphism is a morphism of lie algebras that is a linear. All matrices m.
A lie algebra homomorphism is a linear map φ. Since the theory of finite groups is complicated enough it makes. And the classification as found by killing and cartan the list of all semisimple lie algebras consists of 1 the special linear ones i.
Usually we denote lie algebras by small german letters.
Pdf Simple Singularities And Simple Lie Algebras
Untitled
Pdf A Note On Derivations Of Lie Algebras
Lie Algebras Maths14s Blog
Institutul De Matematica Al Academiei Romane Bucu Re9ti
Lie Algebras And Applications Francesco Iachello Springer
Seminar On Quantum Integrable Models Jules Lamers
Humphreys Introduction To Lie Algebras And Representation
W X Tm Y Tr M X Y R8r Mxlyc Tr D Tmd
Affine Lie Algebras The Symmetric Groups And
Figure 1 From Notes On G2 The Lie Algebra And The Lie
Lie Algebra Representation Wikipedia
